Note : Les descriptions sont présentées dans la langue officielle dans laquelle elles ont été soumises.
CA 02905668 2015-09-25
1
Method and system for determining core losses in a permanent magnet
synchronous motor
Field of the invention
The present invention relates to permanent magnet synchronous
motors, and more precisely to the determination of core losses in a
permanent magnet machine synchronous motor.
Description of the Related Art
Permanent magnet machines and surface mounted permanent
magnet synchronous motors are well suited for high speed applications,
which require robust rotors.
New efficiency standards are likely to be applied to permanent
magnet synchronous motors in the upcoming years. These standards
require low levels of losses. By way of example, a new IES Premium
Efficiency European Standard could be applied to permanent magnet
synchronous motors coupled with variable frequency drives.
It is therefore needed to determine the losses in a permanent
magnet synchronous motor.
It has thus been proposed to directly test the motors in use,
namely when the motors are connected to a load. These methods,
frequently named direct methods and described in the IEC 60034-2, IEE
115 and NEMA standards, may not be suitable for mid to high power
testing of high speed motors because of complexity issues and the
expensiveness of power supplies and cooling equipment.
Indirect methods have also been proposed. Known methods
include the segregated losses method. The IEC 60034-29 standard
summarizes the various indirect testing methods for different motors
(induction motors, DC motors and Synchronous motors).
It should be noted that these methods do not apply strictly to
permanent magnet synchronous motors, but the retardation and short
CA 02905668 2015-09-25
=
2
circuit methods of the IEC 60034-2 standard may still be used for the
permanent magnet synchronous motors.
It has been observed that permanent magnet synchronous motors
present a non-negligible armature reaction due to the high air-gap, more
precisely due to the mechanical air-gap for cooling and the retaining
sleeve. The estimation of core losses when no load is connected to the
motor is consequently wrong using the methods of the prior art.
A method according to the prior art to determine core losses may
comprise:
- A retardation or deceleration or coast-down test in vacuum and
under different air pressure levels in order to deduce windage
losses,
- A retardation or deceleration or coast-down test in vacuum and
under different current biases in order to deduce the rotor active
magnetic bearing losses,
- A retardation or deceleration or coast-down test under atmospheric
pressure to deduce the total no-load losses,
- A deduction from the total no-load losses of the rotor active
magnetic bearings losses and the windage losses.
The deduction step only provides a no-load value, which may be
used with the following equation to obtain the core losses Paw, when a
load is connected to the motor:
( 1 1
PCore 'VE20
REddy RHyst. f I
With REddy representing the quantity of Eddy currents, and RHyst
representing the hysteresis quantity, f the frequency of the motor, Vs0 the
no-load back electromotive force.
According to the prior art, it is possible to use the frequency and
electromotive force corresponding to a situation where a load is connected
to obtain the core losses.
However, it has been observed that R
¨Eddy and RHyst vary with
respect to the flux density level. This is caused by the armature reaction
of permanent magnet synchronous motors. Consequently, the no-load
core losses are useless to determine the full load core losses.
It is a primary object of the invention to provide methods and
system that overcome the deficiencies of the currently available systems
and methods.
CA 02905668 2015-09-25
,
3
Summary of the invention
The present invention overcomes one or more deficiencies of the
prior art by proposing a method for determining core losses in a
. permanent magnet synchronous motor, comprising a measurement of the
losses of the motor with the motor not connected to a load, and a
deduction of the core losses of the motor from the losses of the motor.
According to a general aspect of the method, the motor is placed
in an over-fluxing state during the measurement of the losses.
The inventors have observed that by placing a motor in an over-
fluxing state, with the motor being in a no-load condition (not connected
to a load), the flux density in the motor is close to the flux density of the
motor connected to a load. The determination of the core losses therefore
takes the armature reaction into account and is more accurate.
According to a specific embodiment, the method comprises
placing a filter between the motor and an electric power supply, the filter
being configured to supply reactive current to the motor.
By supplying reactive current to the motor, its magnetic state is
close to the one of a motor connected to a load, and the remaining active
currents that are consumed by the motor correspond to losses.
It is not required to use the above mentioned formula of the prior
art because the obtained core losses are the same as the ones when the
motor is connected to a load: there is no extrapolation.
According to a specific embodiment, the filter is a LC filter.
By simply adding an LC filter between a power supply and the
motor, mainly reactive currents may be fed to the motor.
According to a specific embodiment, a direct component
Ioverfluxing of the current supplied to the motor for placing the motor in
said over-fluxing condition obtained by the following equation:
x
I overfluxing ' II2 'load + B k 2 2. inz
II
j d=13 a
a k m .COSCIT ¨ 0),
with 'load being a current associated with a load, Bm the flux density due
to the magnets of the motor, k a geometrical coefficient, 13,,õ the
maximum flux density of the motor and 9 a phase shift.
CA 02905668 2015-09-25
4
According to a specific embodiment, the deduction of the core
losses comprises a determination of at least one type of losses among
copper losses, time harmonic losses, active magnetic bearing losses,
windage losses, and Litz copper losses.
Again, it should be noted that it is not required to use the above-
mentioned equation of the prior art to obtain the core losses, because
they may be obtained from the total losses after the other losses have
been subtracted.
The invention also proposes a system for determining core losses
in a permanent magnet synchronous motor, comprising means for
measuring the losses of the motor with the motor not connected to a load,
and means for deducting the core losses of the motor from the losses of
the motor.
According to a general aspect of the system, the system
comprises means for placing the motor in an over-fluxing state during the
measurement of the losses.
According to a specific embodiment, the means for placing the
motor in an over-fluxing state comprise a filter between the motor and an
electric power supply, the filter being configured to supply reactive current
to the motor.
According to a specific embodiment, the filter is a LC filter.
According to a specific embodiment, a direct component
lover f luxing of the current supplied to the motor for placing the motor in
said over-fluxing condition obtained by the following equation:
sz .) 11 ad=Bmax
overfluxing \1112oad kmz k 'cosar ¨ 19),
with /load being a current associated with a load, Bõ, the flux density due
to the magnets of the motor, k a geometrical coefficient, Bina, the
maximum flux density of the motor and 6 a phase shift.
According to a specific embodiment, the means for deducting the
core losses of the motor comprise means for determining at least one type
of losses among copper losses, time harmonic losses, active magnetic
bearing losses, windage losses, and Litz copper losses.
4a
In accordance with an aspect of an embodiment, there is
provided a method for determining core losses in a permanent magnet
synchronous motor, the method comprising: obtaining, by at least one
power analyzer, a measurement of the losses of the permanent magnet
synchronous motor with the permanent magnet synchronous motor not
connected to a load, and a deduction of the core losses of the permanent
magnet synchronous motor from the losses of the permanent magnet
synchronous motor; and utilizing a filter between the permanent magnet
synchronous motor and an electric power supply to enable an over-fluxing
state during the measurement of the losses of the permanent magnet
synchronous motor, the filter being configured to supply reactive current
to the permanent magnet synchronous motor.
In accordance with another aspect of an embodiment, there is
provided a system for determining core losses in a permanent magnet
synchronous motor, the system comprising: the permanent magnet
synchronous motor; a measuring device for measuring the losses of the
permanent magnet synchronous motor with the permanent magnet
synchronous motor not connected to a load; a feature for deducting the
core losses of the permanent magnet synchronous motor from the losses
of the permanent magnet synchronous motor, a motor operating
controller comprising a filter, the motor operating controller enabling the
permanent magnet synchronous motor in an over-fluxing state during the
measurement of the losses, the filter being configured to supply reactive
current to the permanent magnet synchronous motor and being located
between the permanent magnet synchronous motor and an electric
power supply.
Date recue / Date received 2021-12-14
CA 02905668 2015-09-25
Brief description of the drawings
How the present invention may be put into effect will now be
described by way of example with reference to the appended drawings, in
5 which:
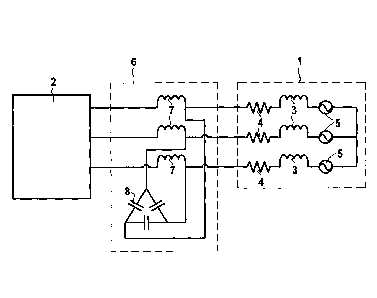
- figure 1 is a schematic representation of an arrangement of a motor, a
filter, and a power supply,
- figure 2 is a diagram of the flux in a motor connected to a load,
- figure 3 is a diagram of the flux in an over-fluxing state,
- figure 4 is a d-q diagram of the motor in an over-fluxing state,
- figures 5 and 6 are representations of the flux density when the motor is
connected to a load and when the motor is in an over-fluxing state,
- figures 7 and 8 are diagrams of the flux density when a load is
connected to a motor, when the motor is not connected to a load, and
when the motor is placed in an over-fluxing state,
- figure 9 is a spectral analysis.
Description of the embodiments
On figure 1, a permanent magnet synchronous motor 1 having
three phases A, B and C has been represented. The motor 1 is a 300
kilowatt and 20500 revolutions per minute motor.
The motor 1 receives electrical power produce by an electric
power supply 2, here a variable frequency drive. The electric power supply
2 provides current for the three phases A, B and C of the permanent
magnet synchronous motor 1.
It should be noted that on figure 1, the motor 1 is not connected
to a load and only its stator has been represented. For each phase A, B or
C, the permanent magnet synchronous motor 1 has a winding 3
represented with its intrinsic resistor 4 and a voltage source 5
corresponding to the no-load electromotive force.
In order to place the permanent magnet synchronous motor 1 in
an over-fluxing state, a filter 6 is placed between the motor 1 and the
electric power supply 2. The filter 6 is configured to supply reactive
current to the motor (without taking into account the parasitic resistors of
CA 029056682015-09-25
6
the filter). In order to do so, the filter 6 is a LC filter comprising for
each
phase a self 7 connected in series between the electric power supply 2
and the motor 1, and a capacitor 8 having an electrode connected
between the self 7 and the motor 1 and an electrode common to another
capacitor.
In order to test the motor at least one power analyzer is
connected to the motor. Preferably, two power analyzers are used for
redundancy and in order to minimize the error in the measurement. The
power analyzers may also measure the total harmonic distortion current,
which may preferably be kept under 5% during the measurements.
Additional means may also be used to determine the total losses.
After the motor has been placed in an over-fluxing state, a
deduction of the core losses may be carried on by determining all the
other types of losses through methods known to the skilled man.
More precisely, the deduction of the core losses may comprise a
determination of at least one type of losses among copper losses, time
harmonic losses, active magnetic bearing losses, windage losses, and Litz
copper losses.
Figures 2 to 4 will explain how the required level of current is
obtained.
Figure 2 is a diagram of the flux in a motor connected to a load,
wherein the flux in the air-gap is represented by a vector B
- airgap= When
the motor is connected to a load, Bairgap is the resultant of two
components, the flux density due to the magnets of the motor Bm and the
armature reaction kiload. The direction of the resultant Bairgap iS
determined by the working conditions of the motor and by the angle 0
between Bm and k. 'load =
By way of example, for maximum torque, Bm and k./(õd are in
quadrature (0 = n/2).
When no load is connected or coupled to the motor, in order to
obtain the same flux density level in the air-gap, the motor has to be
placed in an over-fluxing state. This requires that the magnet flux density
Bin be collinear to the armature reaction flux density k. 'toad (figure 3).
The level of over-fluxing current, here its direct component, may
then be deduced from the following equation:
B2 II d.Bnia
loverfluxing -1112oad ' kmz 2. a k x .COS(TU ¨ 0),
CA 02905668 2015-09-25
7
with 'load being a current associated with a load, Bm the flux density due
to the magnets of the motor, k a geometrical coefficient, Bmax the
maximum flux density of the motor and 0 the phase shift.
The coefficient k is determined by notably taking into account the
number of turns of the windings, the internal stator diameter, the length
of the motor, the winding arrangements, the air-gap length.
It should be noted that loverfluxing is lower than the corresponding
load current with the motor connected to a load.
Figure 4 is a d-q diagram (direct-quadrature diagram using Park's
transformation) showing the motor in an over-fluxing state.
On this figure, Vs is the motor voltage at the end box, and Eci is
the no-load electromotive force.
As can be seen on the figure, a small resistive voltage drop
R. Ioverfluxing can be observed due to the resistive losses. These losses
only correspond to the remaining active power that reaches the motor.
Figures 5 is a representation of the flux density in the motor
connected to a load, with different shadings to highlight the level of flux
density in teslas. On this figure, the dark areas 10 and 11 respectively
correspond to highest levels of flux in the stator and in the air-gap.
By placing a motor in an over-fluxing state, without connecting it
to a load, a similar distribution of flux may be observed in the motor
(figure 6). It should be noted that the two areas 12 and 13 in which the
density is at its highest level are in quadrature.
Figures 7 and 8 are diagrams showing the flux density B
¨ airgap
along the air-gap (from 0 to 360 ).
More precisely, on figure 7, the flux density Bairgap of a motor
not connected to a load and the flux density of a motor connected to a
load are represented. As can be seen on this figure, The two flux densities
differ.
On figure 8, the flux density B
¨ airgap of a motor in an over-fluxing
state and of a motor connected to a load are represented. The two flux
densities appear to be similar. A spectral analysis of the flux density may
be used to confirm this similarity, especially for the lower space
harmonics.
Figure 9 is a spectral analysis of the air gap flux density that
confirms the similar behaviors of the of a motor in an over-fluxing state
and of a motor connected to a load with respect to the no-load situation.
CA 02905668.2015-09-25
8
As can be seen on this figure, for lower space harmonics, the behavior is
similar.